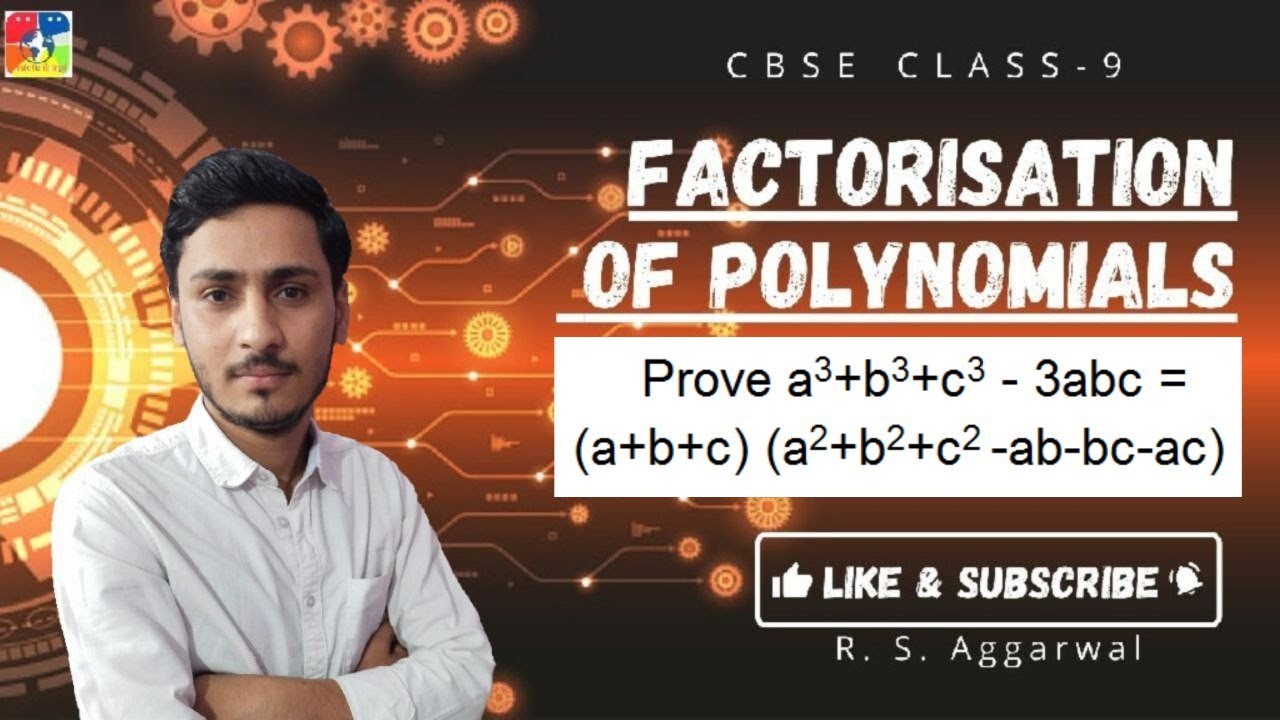
A 2 B 2 3 B 2 C 2 3 C 2 A 2 3 Factorise

Tap for more steps. Can you please help me to factorize the following 1 a 2 b c b 2 c a c 2 a b 2abc 2. Algebra polynomials and rational expressions solution.

1 to 1 2 pvc reducer coupling 1 rod 1 reel fishing 1 utama to klia2 1 2 3 go hindi 1 tahun sama dengan berapa hari 1 ekar berapa meter square 1 phone realme 5 1 to 1 2 copper reducer

Either a or b and for any n if 3 does not divide.

A 2 b 2 3 b 2 c 2 3 c 2 a 2 3 factorise. Equation at the end of step 3. Rewrite using the commutative property of multiplication. Multiply the exponents in.

Tap for more steps. N then n 2 1 mod3 which forces a 2 1mod3. 3 a 4b 2 a 2c 4 a 4c 2 b 4c 2 a 2b 4 b 2c 4 if you rearange the terms in the last factor you will see that they are identical to the result we got form multiplying the left hand side hence.

A 2 b a 2 c ab 2 ac 2 b 2 c bc 2 thoughtfully split the expression at hand into groups each group having two terms. A 2 b c b 2 c a c 2 a b step 4. Use the binomial theorem.

If 3 does not divide ab then 3 does not divide. Apply the power rule and multiply exponents. A 2 b a 2 c group 3.

B 2 c ab 2 group 2. And b 2 1mod3 and c 2 a 2 b 2 1 1 2. Ac 2 bc 2 pull out from each group.

Use factor theorem to prove x y z 3 y log on. A 2 c 3 a 3 c 2. A 3 b 2 a 3 c 2 a 2 b 3 a 2 c 3 b 3 c 2 b 2 c 3 thoughtfully split the expression at hand into groups each group having two terms.

Square formulas a b 2 a2 b2 2ab a b 2 a2 b2 2aba2 b2 a b a b x a x b x2 a b x ab a b c 2 a2 b2 c2 2ab 2bc 2ca. Mod3 but c 2 2 mod3 is impossible. Trying to factor by pulling out.